Mathematics 446
The history of mathematics - Spring, 2005
- Instructor: Bill Casselman
- Class times: MWF 1:00 - 2:00
- Class location: Buchanan B313
- Office hours: by appointment only.
- Email address: cass@math.ubc.ca. I usually respond to email within a few hours.
- Office: Mathematics 215
NOTE: there will be no class Wednesday, January 19. Class will take place on Friday.
This term the main topic will be the history of numbers (as opposed to geometry). The course will be basically a series of snapshots of different developments. We'll begin with early ways of representing numbers, and then look at Euclid's treatment of both magnitudes and integers. Eventually we'll look at Dedekind's treatise on real numbers, the ultimate development of Book V of Euclid's Elements.
References will be scattered - some in libraries, many on the Internet. All students will be expected to read and digest original sources (translated into English). They will also be expected to do independent projects, mostly involving an essay explaining what they find in an original source. Class notes will apppaer on this site from time to time. Some idea of what the course will be like can be seen at the web page for Math 446 - 2003.
Every student taking this course must have previously taken 27 units of courses in either mathematics or computer science. If the credits are in computer science they must be approved by me explicitly.
Grading will be based on (1) roughly weekly assignments; (2) exams - a mid-term examination, a final examination, and numerous quizzes; (3) a final essay project. As a rule, every day on which an assignment is due there will be a short quiz on the same material. Exams and quizzes will be graded proportionally to duration. I have not yet decided how the three different components will be weighted. More information about the essay projects will be posted here later on.
In order not to get a grade of 0 on a quiz or examination, a student must present a valid medical excuse within 2 days of the missed exam, or arrange the absence, and have it approved by the insructor, at least 2 days in advance.
Texts
- Greek & Latin text of The measurement of the circle
- Heath's discussion of Bhaskara's work (from Diophantus)
- H. J. Smith's article on continued fractions (New more compact version posted 9:00 PM Thursday, March 10.)
- Your responsibility in reading Smith's paper is to understand how to read off the continued fraction and related data from the string of letters and how to get the string from the continued fraction.
- Dedekind's article on real numbers
- My notes on Dedekind's article (POSTED THURSDAY 6:00)
- The last reading assignment on decimal fractions (from the JSTOR site, a paper published in 1768)
Homework assignments
- First assignment (due next Monday, January 10 in class!)
- Second assignment (due next Monday, January 17 in class) (solutions)
- Third assignment (due next Monday, January 24 in class) and the copy of the tablet you need. (solutions)
- Fourth assignment (due next Monday, January 31 in class) [posted 21:16 Thursday PM] (solutions)
- Fifth assignment (due next Monday, February 7 in class) [posted 20:05 Wednesday (Ground hog day)] (solutions)
- Sixth assignment (due next Wednesday, February 23 in class)
- Seventh assignment (due next Wednesday, March 2 in class)
- Eighth assignment (due next Wednesday, March 9 in class)
- Ninth assignment (due next Friday, April 1 in class)
- Some points about studying Dedekind's article
- Be sure you know exactly the definition of a cut. In the following sequence of exercises, use only properties of rational numbers and the definition of cut, or something already proven. Be sure everything you refer to has been defined.
- If r is a rational number and (A,B) a cut then A-r is the set made up of a-r and B-r is the set of b-r for a and b in A, B. Show (A-r,B-r) is also a cut.
- Prove that if (A,B) is a cut and r a positive rationl then A-r is contained in A.
- Prove that if (A,B) is a cut and r a positive rationl then there exists s in A with s+r in B.
- Prove that if xi is an increasing sequence of rational numbers that is bounded, then there exists a cut representing the limit. Define this cut x, then show that given any positive rational e there exists N such that x-e is less than xi for i greater than N.
- If x = (A,B) is a cut representing a positive real number, define the cut corresponding to x2.
- Define 2 as a cut.
- Define the square root of 2 nas a cut.
- Prove that the square of the square root of 2 is 2.
The projects
Every student is required to hand in a project at the end of the term. These will normally be essays of some kind explaining something about mathematics and history. All project topics must be approved by the instructor. An essay should normally be about 20-30 pages in length, and done by computer word-processing. Illustrations are encouraged. All sources - all illustrations and text not produced entirely by you - must be acknowledged. Grades will be based on:- Mathematical content
- Historical content
- Relevance to course material
- Difficulty
- Interest
- Originality
- Clarity
- Accuracy
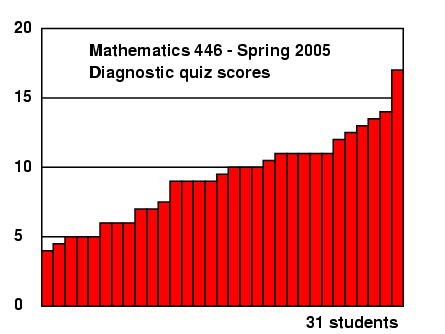
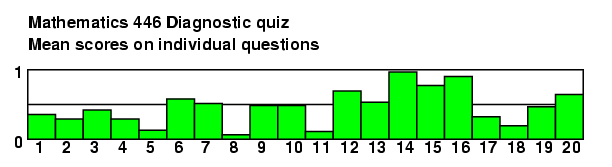
The diagnostic quiz
- The quiz itself
-
Grade distributions: