|
Scaling and Shifting |
Basic properties of Wave Functions |
Wave Equations |
Affine Functions |
Waves in 2-D and 3-D |
|
Scientists believe that light travels as waves. Light behaves in a similar way to waves in rope and in water. Let us take a look at the 4 basic properties for waves -
Wavelength: Wavelength (also known as lambda - 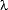 ) is defined as the distance from one crest (or maximum of the wave) to the next crest or maximum. To be more specific, a wavelength exists between any point on a wave and the corresponding point on the next wave in the wave train, which can be seen below:
) is defined as the distance from one crest (or maximum of the wave) to the next crest or maximum. To be more specific, a wavelength exists between any point on a wave and the corresponding point on the next wave in the wave train, which can be seen below:
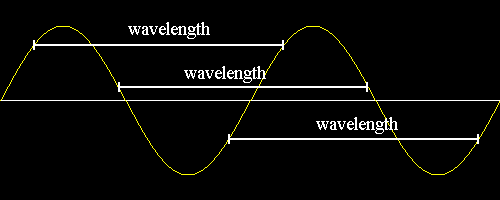
Unit: any metric unit of length (m, cm, mm, etc.).
It is interesting to know that the color of light depends on its wavelength. Visible light has wavelengths from ~400-700 nanometers long. The 400 nm light (higher energy) is blue, the 700 nm light is red, and the various colors fall in between the two. Further details later on.
Amplitude: The height of the wave is called its amplitude. We measure the middle of the wave to its peak as THE amplitude.
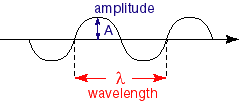
Unit: Because amplitude is a distance measure, any metric unit of length or distance is appropriate for amplitude (m, cm, mm, etc.).
Velocity: The velocity of the wave is the measurement of how fast a crest is moving from a fixed point. For example, the velocity of water waves can be measured as their speed in a given direction with respect to the land. As for light waves, the velocity is equal to the speed of light, c, (2.998*10^8 m/s)
Unit: any metric unit of length per time units (m/s).
Frequency:
The frequency of waves  , is the rate the crests or peaks pass a given point per second. If the period is 1/2 second, the frequency will be two wavelengths per second (1/2 second for one wavelength, so two wavelengths in one second). The greater the wavelength the lower the frequency. While the lower the frequency, the higher the wavelength. Frequency is the wavelength divided by the velocity.
, is the rate the crests or peaks pass a given point per second. If the period is 1/2 second, the frequency will be two wavelengths per second (1/2 second for one wavelength, so two wavelengths in one second). The greater the wavelength the lower the frequency. While the lower the frequency, the higher the wavelength. Frequency is the wavelength divided by the velocity.
Unit: Cycles per second (a.k.a. Hertz).
Frequency = Velocity / Wavelength
Another way of writing that is:
Velocity = Wavelength x Frequency
T=period=1/( /2
/2 ) = 2
) = 2 /
/
Suppose the time period is 10 seconds, the frequency would be 1/10 of a period per second.
A mini exercise
Since this is a math-related webpage, we would like to perform a very simple calcuation:
Example: What is the wavelength of a light wave with frequency 3.25*10^6 /s?
Solution: Simply use the relationship between the frequency and wavelength of a light wave -
 = c/
= c/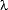
Solving this for the wavelength gives
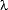 = c/
= c/
so we have
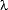 = 2.998*10^8 m/s / (3.25*10^6/s)
= 2.998*10^8 m/s / (3.25*10^6/s)
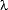 = approximately 0.92 meters.
= approximately 0.92 meters.
By: Edmund Lai and Leo Cheng
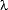 ) is defined as the distance from one crest (or maximum of the wave) to the next crest or maximum. To be more specific, a wavelength exists between any point on a wave and the corresponding point on the next wave in the wave train, which can be seen below:
) is defined as the distance from one crest (or maximum of the wave) to the next crest or maximum. To be more specific, a wavelength exists between any point on a wave and the corresponding point on the next wave in the wave train, which can be seen below:


 , is the rate the crests or peaks pass a given point per second. If the period is 1/2 second, the frequency will be two wavelengths per second (1/2 second for one wavelength, so two wavelengths in one second). The greater the wavelength the lower the frequency. While the lower the frequency, the higher the wavelength. Frequency is the wavelength divided by the velocity.
, is the rate the crests or peaks pass a given point per second. If the period is 1/2 second, the frequency will be two wavelengths per second (1/2 second for one wavelength, so two wavelengths in one second). The greater the wavelength the lower the frequency. While the lower the frequency, the higher the wavelength. Frequency is the wavelength divided by the velocity.
 ) = 2
) = 2